Uncertainty explained: How is a calibration result reached?

By doing that, we can better identify the parameters which can have a possible influence on a calibration result, and this becomes vital in order to create an uncertainty budget.

Read along, while we explore the importance of specific factors and measurements from an uncertainty perspective.

> Uncertainty explained: Understanding an uncertainty budget.

The technical part: How do calibration laboratories reach a calibration result?

When calculating uncertainty, one starts with a model equation. So, we start by asking the question: How do calibration laboratories reach a calibration result? That is, how do you calculate the error of a meter under test (MUT)? 

To do this, we take a top down approach and start with the basics of how to calculate the calibration result or error of a MUT. A calibration result can be calculated based on flow or volume with the following equation:

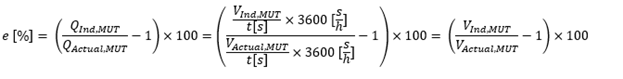
In the following calculations, we assume an accurate enough signal collection system, that the signal collection starts and stops at the same time for both the Meter Under Test (MUT) and the working standards (WS) used to calibrate the MUT. Thus, subsequent calculations will all be done based on volume.

Here, laboratories normally use reference meters, also called working standards or (WS). These WS have themselves been calibrated and are set up in series with the MUT. By using these meters, and continuously collecting data from the WS, calibration laboratories know the gas flow through the WS at all times, although of course with an uncertainty, but we will come back to that later.

But to generate flow in a system, a pressure difference is generated and there might also be a temperature difference between the WS and MUT, so knowing the gas flow rate at the WS’s is not enough. This is where the “ideal” gas law comes in. However, as we are not working with ideal gasses, we add the compressibility factor to the ideal gas law equation.

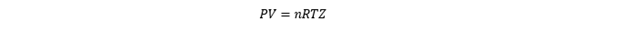
And if there is no pressure leakage in or out, and the amount of gas stays the same between the WS and the MUT, we should get the same mass flow trough the WS and the MUT. Expressed in another way, we should have the same amount of mol (n) through the meter under test and the working standard.

So, for calibration laboratories this means that if there are two positions 1 and 2, where gas flows through both positions and there is no loss or gain of gas between the positions, then the same amount of mol flows through the two positions.

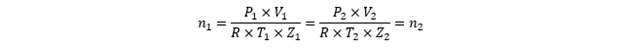
Using this condition, we can now isolate V₁ on one side of the equation and instead of calling it position 1 and 2, we say that these two positions are the positions of the WS and the MUT in the calibration loop. Using this, we can now isolate V₁=V_MUT on one side of the equation. Since both sides of the equation divide by the constant R, it falls out and we get:

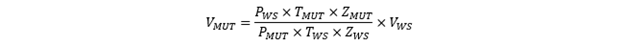
Since the WS is itself a calibrated meter, we can obtain a V_Actual, _MUT that is traceable to the WS if we correct for the error of the WS, based on its calibration. So, we get:

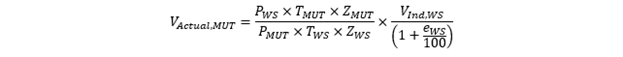
We now have all the elements that are used to calculate the error of a MUT in a calibration, so the model equation (equation 1) can now be expanded upon so:

Using this expanded form of the model equation, we have all the factors needed to calculate uncertainty in a calibration.

Want to know more about uncertainty and calibration results?

• We are always open for discussions - contact us.
  
  
• Sign up to receive news and articles about gas flow calibration
  
  
• Read more: Uncertainty explained - Class Half meters
  
  
• Learn more about Calibration here.

Symbols

The equations used in this article use symbols that are based on what is normally used in international standards and documents.

Table 1: Symbols and their description.